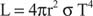The determination of the radius of a star requires observation of its angular size, which with its distance yields the star's true linear size. However, because stars are so distant, they appear to be only a fraction of an arc second across, an apparent diameter that is vastly increased by the blurring effects of the atmosphere. The apparent diameter can also be increased by the diffraction effects in a telescope that is in orbit above the atmosphere. There are sophisticated interferometric techniques to measure these tiny angular sizes, but they are difficult to put into practice and not feasible for obtaining large quantities of data. One of these methods is speckle interferometry, a technique in which many short exposure images of a star are recorded at a telescope. The exposure time must be a fraction of a second in order to prevent atmospheric motions from blurring the image, but then each image is insufficiently exposed to reveal any significant detail. Hundreds of images, however, can be combined to form an image with sufficient resolution to determine the angular size of a star and even some detail of the surface features in the case of supergiant stars.
On the other hand, sizes of stars may be more easily obtained by observation of the light coming from eclipsing binaries. If the orbital properties are known, then the length of an eclipse directly gives the size of the eclipsing and eclipsed stars.
More generally, a stellar radius R can be directly inferred from use of Stefan‐Boltzman's Law if the surface temperature T and luminosity L are known for a star, that is, from the relationship