The study of numerical data and their distribution is called statistics.
Any measure indicating a center of a distribution is called a measure of central tendency. The three basic measures of central tendency are
- Mean (or arithmetic mean): Usually called the average.
- Median: The middle number in a set of numbers arranged in ascending or descending order.
- Mode: The set, class, or classes that show up most often.
Mean
The mean (arithmetic mean) is the most frequently used measure of central tendency. It is generally reliable, easy to use, and more stable than the median. To determine the arithmetic mean, simply total the items and then divide by the number of items.
Example 1
What is the arithmetic mean of 0, 12, 18, 20, 31, and 45?
Total the items.
0 + 12 + 18 + 20 + 31 + 45 = 126
Divide by the number of items.
126 ÷ 6 = 21
The arithmetic mean is 21.
Example 2
What is the arithmetic mean of 25, 27, 27, and 27?

The arithmetic mean is  .
.
Example 3
What is the arithmetic mean of 20 and –10?
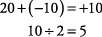
The arithmetic mean is 5.
When one or a number of items is used several times, those items have more “weight.” This establishment of relative importance, or weighting, is used to compute the weighted mean.
Example 4
What is the mean of three tests averaging 70% plus seven tests averaging 85%?
In effect, you have ten exams, three of which score 70% and seven of which score 85%. Rather than adding all ten scores, to determine the above “weighted mean,” simply multiply 3 times 70% to find the total of those items (210). Then multiply 7 times 85% to find their total (595). Now add the two totals (805) and divide by the number of items overall (10). The weighted mean is thus 80.5%.
Example 5
For the first nine months of the year, the average monthly rainfall was 2 inches. For the last three months of that year, rainfall averaged 4 inches per month. What was the mean monthly rainfall for the entire year?
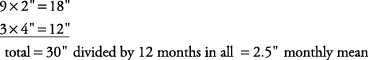
Example 6
Six students averaged 90% on a class test. Four other students averaged 70% on the test. What was the mean score of all ten students?
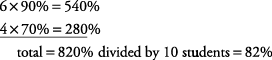
Median
The median of a set of numbers arranged in ascending or descending order is the middle number if there is an odd number of items in the set. If there is an even number of items in the set, their median is the arithmetic mean of the middle two numbers. The median is easy to calculate and is not influenced by extreme measurements.
Example 7
Find the median of 3, 4, 6, 9, 21, 24, 56.
3, 4, 6, 9, 21, 24, 56
The median is 9.
Example 8
Find the median of 4, 5, 6, 10.
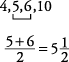
The median is  .
.
Mode
The set, class, or classes that appear most, or whose frequency is the greatest, is the mode or modal class. (Mode is not greatly influenced by extreme cases but is probably the least important or least used of the three measures of central tendency.)
Example 9
Find the mode of 3, 4, 8, 9, 9, 2, 6, 11.
The mode is 9 because it appears more often than any other number.