In each case, the work done is the area under the curve. Note that in Figure (d), the area under the curve is zero; no work is done in the isochoric process.
The engineer N. L. Sadi Carnot (1796–1832) first proposed an ideal heat engine that operated through a cycle of reversible isothermal and adiabatic steps. Imagine the engine to be an idealized gas in a cylinder with a fitted piston that supports a load as shown in Figure 3. During four steps on one down and upward stroke of the piston, visualize the gas and cylinder sitting first on a heat source (heat is added), then on an insulator (no heat exchange), next on a heat sink (heat is removed), and finally back on the insulator.
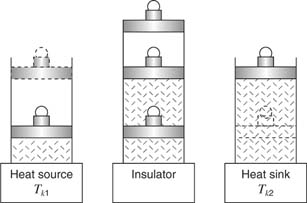
Figure 3
The Carnot cycle.
The pressure‐volume curve of Figure shows the Carnot cycle. The gas in the cylinder contains an ideal gas at pressure (P), volume (V), and temperature (T)—point A on the curve. The cylinder with gas is set on a heat source and expands isothermally (the temperature remains constant as the pressure decreases and the volume increases) to point B on the graph. During this isothermal expansion, the gas did work lifting a load (or turning a wheel). This work is represented by the area under the A–B curve between V 1 and V 2. Now, the gas and cylinder are placed on an insulator; the gas expands adiabatically (no heat exchange with the outside world) to point C on the curve. More work is done by the gas on the piston through this expansion, represented by the area under the B–C curve between V m and V 3.
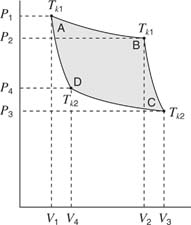
Figure 4
P‐V graph for the Carnot cycle.
Next, the gas and cylinder are placed on a heat sink. The gas is compressed isothermally and gives up an amount of heat to the heat sink. The conditions at point D describe the gas. For this segment, work is done by the piston on the gas, which is represented by the area under the C–D segment of the curve from V 3 to V 4. Finally, the gas and cylinder are placed back on the insulator. The gas is further compressed adiabatically until it returns to the original conditions at point A. Again, for this part of the Carnot cycle, work is done on the gas, which is represented by the area under the D‐A segment between V 4 and V 1.
The total work done by the gas on the piston is the area under the ABC segment of the curve; the total work done on the gas is the area under the CDA segment. The difference between these two areas is the shaded portion of the graph. This area represents the work output of the engine. According to the first law of thermodynamics, there is no permanent loss or gain of energy; therefore, the work output of the engine must equal the difference between the heat absorbed from the heat source and that given up to the heat sink.
Consideration of the work output and input leads to the definition of efficiency of an ideal heat engine. If the energy absorbed from the heat source is Q 1 and the heat given up to the heat sink is Q 2, then work output is given by W output = Q 1 − Q 2. Efficiency is defined as the ratio of the work output over the work input expressed in percent, or

which when expressed in terms of heat is

and in terms of temperature:
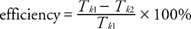
This efficiency is greater than that of most engines because real engines also have losses due to friction.
The second law of thermodynamics can be stated thus: It is impossible to construct a heat engine that only absorbs heat from a heat source and performs an equal amount of work. In other words, no machine is ever 100 percent efficient; some heat must be lost to the environment.
The second law also determines the order of physical phenomenon. Imagine viewing a film where a pool of water forms into an ice cube. Obviously, the film is running backward from the way in which it was filmed. An ice cube melts as it heats but never spontaneously cools to form an ice cube again; thus, this law indicates that certain events have a preferred direction of time, called the arrow of time. If two objects of different temperatures are placed in thermal contact, their final temperature will be between the original temperatures of the two objects. A second way to state the second law of thermodynamics is to say that heat cannot spontaneously pass from a colder to a hotter object.
Entropy is the measure of how much energy or heat is unavailable for work. Imagine an isolated system with some hot objects and some cold objects. Work can be done as heat is transferred from the hot to the cooler objects; however, once this transfer has occurred, it is impossible to extract additional work from them alone. Energy is always conserved, but when all objects have the same temperature, the energy is no longer available for conversion into work.
The change in entropy of a system (Δ S) is defined mathematically as
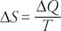
The equation states the following: The change in entropy of a system is equal to the heat flowing into the system divided by the temperature (in degrees Kelvin).
The entropy of the universe increases or remains constant in all natural processes. It is possible to find a system for which entropy decreases, but only due to a net increase in a related system. For example, the originally hotter objects and cooler objects reaching thermal equilibrium in an isolated system may be separated, and some of them put in a refrigerator. The objects would again have different temperatures after a period of time, but now the system of the refrigerator would have to be included in the analysis of the complete system. No net decrease in entropy of all the related systems occurs. This is yet another way of stating the second law of thermodynamics.
The concept of entropy has far‐reaching implications that tie the order of our universe to probability and statistics. Imagine a new deck of cards in order by suits, with each suit in numerical order. As the deck is shuffled, no one would expect the original order to return. There is a probability that the randomized order of the shuffled deck would return to the original format, but it is exceedingly small. An ice cube melts, and the molecules in the liquid form have less order than in the frozen form. An infinitesimally small probability exists that all of the slower moving molecules will aggregate in one space so that the ice cube will reform from the pool of water. The entropy and disorder of the universe increase as hot bodies cool and cold bodies warm. Eventually, the entire universe will be at the same temperature, so the energy will be no longer usable.