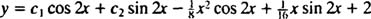In order to give the complete solution of a nonhomogeneous linear differential equation, Theorem B says that a particular solution must be added to the general solution of the corresponding homogeneous equation.
If the nonhomogeneous term d( x) in the general second‐order nonhomogeneous differential equation
is of a certain special type, then the method of undetermined coefficientscan be used to obtain a particular solution. The special functions that can be handled by this method are those that have a finite family of derivatives, that is, functions with the property that all their derivatives can be written in terms of just a finite number of other functions.
For example, consider the function d = sin x. Its derivatives are
and the cycle repeats. Notice that all derivatives of d can be written in terms of a finite number of functions. [In this case, they are sin x and cos x, and the set {sin x, cos x} is called the family (of derivatives) of d = sin x.] This is the criterion that describes those nonhomogeneous terms d( x) that make equation (*) susceptible to the method of undetermined coefficients: d must have a finite family.
Here's an example of a function that does not have a finite family of derivatives: d = tan x. Its first four derivatives are
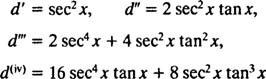
Notice that the nth derivative ( n ≥ 1) contains a term involving tan n‐1 x, so as higher and higher derivatives are taken, each one will contain a higher and higher power of tan x, so there is no way that all derivatives can be written in terms of a finite number of functions. The method of undetermined coefficients could not be applied if the nonhomogeneous term in (*) were d = tan x. So just what are the functions d( x) whose derivative families are finite? See Table 1.
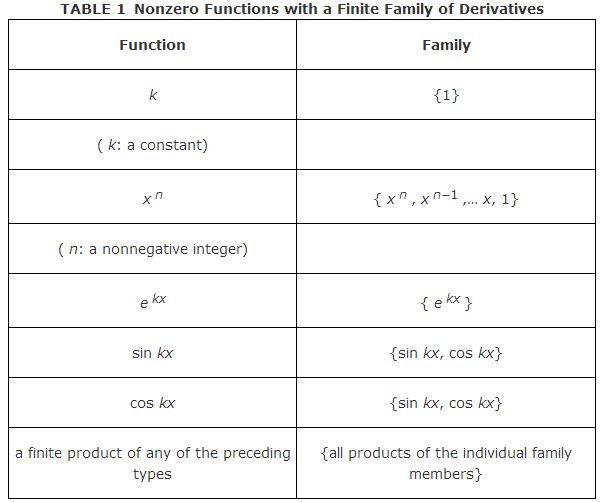
Example 1: If d( x) = 5 x 2, then its family is { x 2, x, 1}. Note that any numerical coefficients (such as the 5 in this case) are ignored when determining a function's family.
Example 2: Since the function d( x) = x sin 2 x is the product of x and sin 2 x, the family of d( x) would consist of all products of the family members of the functions x and sin 2 x. That is,
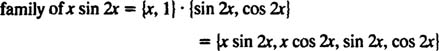
Linear combinations of n functions . A linear combination of two functions y 1 and y 2 was defined to be any expression of the form
where c 1 and c 2 are constants. In general, a lineral, a linear combination of n functions y 1 y 2,…, y n is any expression of the form
where c 1,…, c n are contants. Using this terminology, the nonhomogeneous terms d( x) which the method of undetermined coefficients is designed to handle are those for which every derivative can be written as a linear combination of the members of a given finite family of functions.
The central idea of the method of undetermined coefficients is this: Form the most general linear combination of the functions in the family of the nonhomogeneous term d( x), substitute this expression into the given nonhomogeneous differential equation, and solve for the coefficients of the linear combination.
Example 3: Find a particular solution of the differential equation
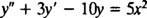
As noted in Example 1, the family of d = 5 x 2 is { x 2, x, 1}; therefore, the most general linear combination of the functions in the family is y = Ax 2 + Bx + C (where A, B, and C are the undetermined coefficients). Substituting this into the given differential equation gives
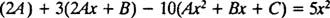
Now, combinbing like terms yields
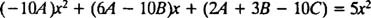
In order for this last equation to be an identity, the coefficients of like powers of x on both sides of the equation must be equated. That is, A, B, and C must be chosen so that
The first equation immediately gives 
. Substituting this into the second equation gives 
, and finally, substituting both of these values into the last equation yields 
. Therefore, a particular solution of the given differential equation is
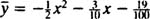
Example 4: Find a particular solution (and the complete solution) of the differential equation
Since the family of d = sin x is {sin x, cos x}, the most general linear combination of the functions in the family is y = A sin x + B cos x (where A and B are the undetermined coefficients). Substituting this into the given differential equation gives
Now, combining like terms and simplifying yields
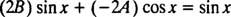
In order for this last equation to be an identity, the coefficients A and B must be chosen so that
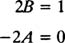
These equations immediately imply A = 0 and B = ½. A particular solution of the given differential equation is therefore
According to Theorem B, combining this y with the result of Example 12 yields the complete solution of the given nonhomogeneous differential equation: y = c 1 e x + c 2 xe x + ½ cos x.
Example 5: Find a particular solution (and the complete solution) of the differential equation
Since the family of d = 8 e −7 x is just { e −7 x }, the most general linear combination of the functions in the family is simply y = Ae −7 x (where A is the undetermined coefficient). Substituting this into the given differential equation gives
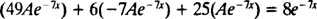
Simplifying yields
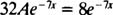
In order for this last equation to be an identity, the coefficient A must be chosen so that 
which immediately gives A = ¼. A particular solution of the given differential equation is therefore 
and then, according to Theorem B, combining y with the result of Example 13 gives the complete solution of the nonhomogeneous differential equation: y = e −3 x ( c 1 cos 4 x + c 2 sin 4 x) + ¼ e −7 x .
Example 6: Find the solution of the IVP
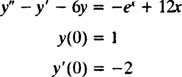
The first step is to obtain the general solution of the corresponding homogeneous equation
Since the auxiliary polynomial equation has distinct real roots,
the general solution of the corresponding homogeneous equation is y h = c 1 e − x + c 2 e 3 x
Now, since the nonhomogeneous term d( x) is a (finite) sum of functions from Table 1, the family of d( x) is the union of the families of the individual functions. That is, since the family of − e x is { e x }, and the family of 12x is { x, 1},
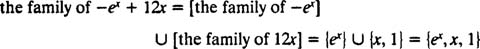
The most general linear combination of the functions in the family of d = − e x + 12 x is therefore y = Ae x + Bx + C (where A, B, and C are the undetermined coefficients). Substituting this into the given differential equation gives
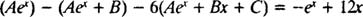
Combining like terms and simplifying yields
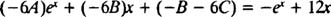
In order for this last equation to be an identity, the coefficients A, B, and C must be chosen so that
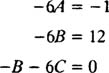
The first two equations immediately give A = ⅙ and B = −2, whereupon the third implies C = ⅓. A particular solution of the given differential equation is therefore
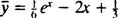
According to Theorem B, then, combining this y with y h gives the complete solution of the nonhomogeneous differential equation: y = c 1 e −2 x + c 2 e 3 x + ⅙ e x –2 x + ⅓. Now, to apply the initial conditions and evaluate the parameters c 1 and c 2:
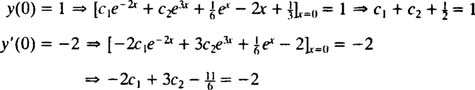
Solving these last two equations yields c 1 = ⅓ and c 2 = ⅙. Therefore, the desired solution of the IVP is
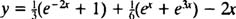
Now that the basic process of the method of undetermined coefficients has been illustrated, it is time to mention that is isn't always this straightforward. A problem arises if a member of a family of the nonhomogeneous term happens to be a solution of the corresponding homogeneous equation. In this case, that family must be modified before the general linear combination can be substituted into the original nonhomogeneous differential equation to solve for the undetermined coefficients. The specific modification procedure will be introduced through the following alteration of Example 6.
Example 7: Find the complete solution of the differential equation
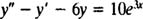
The general solution of the corresponding homogeneous equation was obtained in Example 6:
Note carefully that the family { e 3 x } of the nonhomogeneous term d = 10 e 3 x contains a solution of the corresponding homogeneous equation (take c 1 = 0 and c 2 = 1 in the expression for y h ). The “offending” family is modified as follows: Multiply each member of the family by x and try again.
Since the modified family no longer contains a solution of the corresponding homogeneous equation, the method of undetermined coefficients can now proceed. (If xe 3 x had been again a solution of the corresponding homogeneous equation, you would perform the modification procedure once more: Multiply each member of the family by x and try again.) Therefore, substituting y = Axe 3 x into the given nonhomogeneous differential equation yields
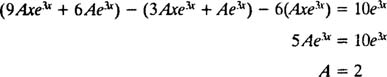
This calculation implies that y = 2 xe 3 x is a particular solution of the nonhomogeneous equation, so combining this with y h gives the complete solution:
Example 8: Find the complete solution of the differential equation
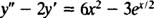
First, obtain the general solution of the corresponding homogeneous equation
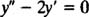
Since the auxiliary polynomial equation has distinct real roots,
the general solution of the corresponding homogeneous equation is
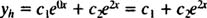
The family for the 6 x 2 term is { x 2, x, 1}, and the family for the −3 e x/2 term is simply { e x/2 }. This latter family does not contain a solution of the corresponding homogeneous equation, but the family { x 2, x, 1} does(it contains the constant function 1, which matches y h when c 1 = 1 and c 2 = 0). This entire family (not just the “offending” member) must therefore be modified:
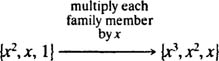
The family that will be used to construct the linear combination y is now the union
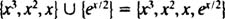
This implies that y = Ax 3 + Bx 2 + Cx + De x/2 (where A, B, C, and D are the undetermined coefficients) should be substituted into the given nonhomogeneous differential equation. Doing so yields
which after combining like terms reads
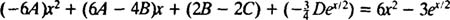
In order for this last equation to be an identity, the coefficients A, B, C, and D must be chosen so that
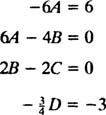
These equations determine the values of the coefficients: A = −1, B = C = 
, and D = 4. Therefore, a particular solution of the given differential equation is
According to Theorem B, then, combining this y with y h gives the complete solution of the nonhomogeneous differential equation: y = c 1 + c 2 e 2 x – x 3 x 2 x + 4 e x/2
Example 9: Find the complete solution of the equation
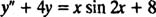
First, obtain the general solution of the corresponding homogeneous equation
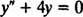
Since the auxiliary polynomial equation has distinct conjugate complex roots,
the general solution of the corresponding homogeneous equation is
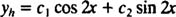
Example 2 showed that the
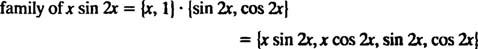
Note that this family contains sin 2 x and cos 2 x, which are solutions of the corresponding homogeneous equation. Therefore, this entire family must be modified:
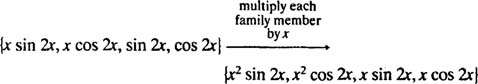
None of the members of this family are solutions of the corresponding homogeneous equation, so the solution can now proceed as usual. Since the family of the constant term is simply {1}, the family used to construct y is the union
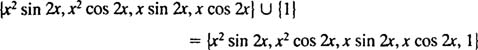
This implies that y = Ax 2 sin 2 x + Bx 2 cos 2 x + Cx sin 2 x + Dx cos 2 x + E (where A, B, C, D, and E are the undermined coefficients) should be substitueted into the given nonhomogeneous differential equation y″ + 4 y = x sin 2 x + 8. Doing so yields
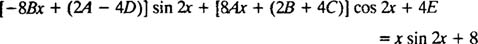
In order for this last equation to be an identity, A, B, C, D, and E must be chosen so that
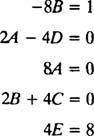
These equations determine the coefficients: A = 0, B = −⅛, C = 
, D = 0, and E = 2. Therefore, a particular solution of the given differential equation is
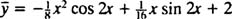
According to Theorem B, then, combining this y with y h gives the complete solution of the nonhomogeneous differential equation:
