Economics

Present value formula. The present value of receiving $20,000 one year from now can be calculated using the present value formula. The formula for finding the present value of X dollars received t years from now at the current market interest rate r is

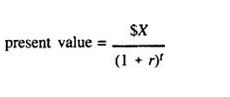
For example, if X = $20,000, t = 1, and r = .05, the present value of $20,000 received one year from now is 20,000/(1.05)¹ = $19,047.62.

The present value of $20,000 received two years from now at an interest rate of 5% is found by setting X = $20,000, t = 2, and r = .05. The present value in this case is $20,000/(1.05)² = $18,140.59. As you can see from these examples, the present value of the future income is the amount of income that you would need to invest today, at current market interest rates, in order to obtain the same amount of future income at the same future date.

Firm's investment decision. The firm's investment decision is to determine whether to purchase new capital. In determining whether to purchase new capital—for example, new equipment—the firm will take into account the price of the new equipment, the revenue that the new equipment will generate for the firm over time, and the scrap value of the new equipment. The firm will also take into account the interest rate, which represents the firm's opportunity cost of investing in the new equipment. It will use the interest rate to calculate the present value of the future net income that it expects to earn from its purchase of the new capital equipment. If the present value is positive, the firm will choose to purchase the new equipment. If the present value is negative, it is better off forgoing the investment in new equipment.

As an example, consider a restaurant that is trying to decide whether to invest in a new piece of capital equipment—a jukebox. The jukebox costs $7,000 and lasts 4 years. The restaurant estimates that the jukebox will provide it with income of $2,000 per year, net of maintenance costs. After 4 years, the scrap value of the jukebox is estimated at $500. In determining whether to purchase the jukebox, the firm will calculate the net present value of the present and future income that it receives from purchasing the jukebox. The firm's present value calculations are shown in Table for an interest rate of 10%.

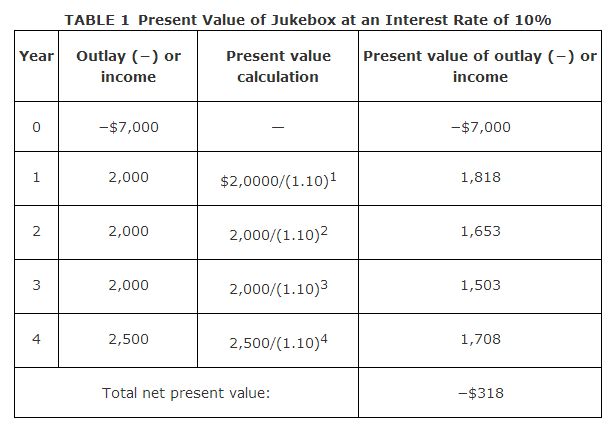
The calculation of net present value includes the initial outlay of $7,000 for the jukebox. The present value formula is used to calculate the present value of the $2,000 annual income received in each of the 4 years. In the fourth year, the $500 scrap value is added to the $2,000 in income received from the jukebox. The total net present value of the jukebox turns out to be −$318. Because this amount is negative, the firm will choose to forgo purchasing the jukebox.

As Table reveals, the firm's net present value calculations depend on the interest rate. The higher the interest rate is, the higher the firm's opportunity cost of investing in the jukebox. A higher interest rate lowers the present value of the future income earned from capital, making it less profitable for the firm to invest in new capital; however, if interest rates fall, the opportunity cost of investing in new capital also falls. The lower the interest rate is, the higher the present value of the future income earned from new capital investment, and the more likely it is that firms will invest in new capital.

For example, consider what happens to the firm's net present value calculations for the jukebox when the interest rate falls from 10% to 6%. Table repeats the present value calculations of Table at the lower interest rate of 6%.

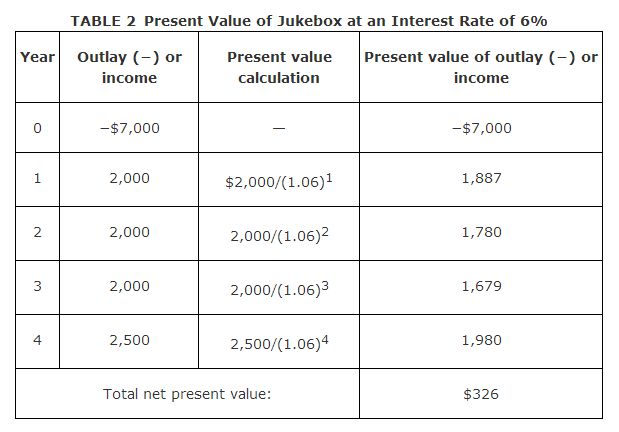
At the lower interest rate, the net present value of the jukebox is positive ($326). If the firm can obtain $7,000 in loanable funds at 6% interest, it will choose to purchase the jukebox.

The decision to invest in other types of capital goods can also be made on the basis of present value calculations. For example, the decision to invest in human capital by attending college is based on the present value of the future income that an individual can earn with a college degree. If the present value is positive, the individual will choose to attend college. If the present value is negative, the individual will not attend college and will perhaps take a job instead.