Consequences of the Parallel Postulate
Postulate 11 can be used to derive additional theorems regarding parallel lines cut by a transversal. Because m ∠1 + m ∠2 = 180 ° and m ∠5 + m ∠6 = 180° (because adjacent angles whose noncommon sides lie on a line are supplementary), and because m ∠1 = m ∠3, m∠2 = m ∠4, m ∠5 = m ∠7, and m ∠6 = m ∠8 (because vertical angles are equal), all of the following theorems can be proven as a consequence of Postulate 11.
Theorem 13: If two parallel lines are cut by a transversal, then alternate interior angles are equal.
Theorem 14: If two parallel lines are cut by a transversal, then alternate exterior angles are equal.
Theorem 15: If two parallel lines are cut by a transversal, then consecutive interior angles are supplementary.
Theorem 16: If two parallel lines are cut by a transversal, then consecutive exterior angles are supplementary.
The above postulate and theorems can be condensed to the following theorems:
Theorem 17: If two parallel lines are cut by a transversal, then every pair of angles formed are either equal or supplementary.
Theorem 18: If a transversal is perpendicular to one of two parallel lines, then it is also perpendicular to the other line.
Based on Postulate 11 and the theorems that follow it, all of the following conditions would be true if l // m (Figure 1).
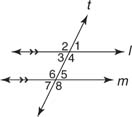
Figure 1 Two parallel lines cut by a transversal.
In figures, single or double arrows on a pair of lines indicate that the lines are parallel.
Based on Postulate 11:
Based on Theorem 13:
Based on Theorem 14:
Based on Theorem 15:
- ∠3 and ∠6 are supplementary
- ∠4 and ∠5 are supplementary
Based on Theorem 16:
- ∠1 and ∠8 are supplementary
- ∠2 and ∠7 are supplementary
Based on Theorem 18:
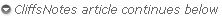
If t ⊥ l, then t ⊥ m