Proving that Figures Are Parallelograms
Many times you will be asked to prove that a figure is a parallelogram. The following theorems are tests that determine whether a quadrilateral is a parallelogram:
Theorem 46: If both pairs of opposite sides of a quadrilateral are equal, then it is a parallelogram.
Theorem 47: If both pairs of opposite angles of a quadrilateral are equal, then it is a parallelogram.
Theorem 48: If all pairs of consecutive angles of a quadrilateral are supplementary, then it is a parallelogram.
Theorem 49: If one pair of opposite sides of a quadrilateral is both equal and parallel, then it is a parallelogram.
Theorem 50: If the diagonals of a quadrilateral bisect each other, then it is a parallelogram.
Quadrilateral QRST in Figure 1 is a parallelogram if:
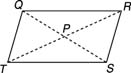
Figure 1 A quadrilateral with its diagonals.
- QR = ST and QT = RS, by Theorem 46.
- m ∠ Q = m ∠ S and m ∠ T = m ∠ R, by Theorem 47.
- ∠ Q and ∠ R, ∠ R and ∠ S, ∠ S and ∠ T, and ∠ Q and ∠ T are all supplementary pairs, by Theorem 48.
- QR = ST and QR ∥ ST or QT = RS and QT ∥ RS , by Theorem 49.
- QP = PS and RP = PT, by Theorem 50.