Triangle Inequalities: Sides and Angles
You have just seen that if a triangle has equal sides, the angles opposite these sides are equal, and if a triangle has equal angles, the sides opposite these angles are equal. There are two important theorems involving unequal sides and unequal angles in triangles. They are:
Theorem 36: If two sides of a triangle are unequal, then the measures of the angles opposite these sides are unequal, and the greater angle is opposite the greater side.
Theorem 37: If two angles of a triangle are unequal, then the measures of the sides opposite these angles are also unequal, and the longer side is opposite the greater angle.
Example 1: Figure 1 shows a triangle with angles of different measures. List the sides of this triangle in order from least to greatest.

Figure 1 List the sides of this triangle in increasing order.
Because 30° < 50° < 100°, then RS < QR < QS.
Example 2: Figure 2 shows a triangle with sides of different measures. List the angles of this triangle in order from least to greatest.
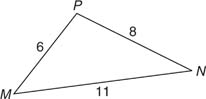
Figure 2 List the angles of this triangle in increasing order.
Because 6 < 8 < 11, then m ∠ N < m ∠ M < m ∠ P.
Example 3: Figure 3 shows right Δ ABC. Which side must be the longest?
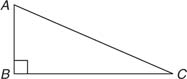
Figure 3 Identify the longest side of this right triangle.
Because ∠ A + m ∠ B + m ∠ C = 180 ° (by Theorem 25) and m ∠ = 90°, we have m ∠ A + m ∠ C = 90°. Thus, each of m ∠ A and m ∠ C is less than 90°. Thus ∠ B is the angle of greatest measure in the triangle, so its opposite side is the longest. Therefore, the hypotenuse, AC , is the longest side in a right triangle.